Paper Square Ball

The Paper Square Ball is part of a family of "slide-together" paper constructions that are made by cutting a number of identical pieces of paper which fit together without tape or glue. In this construction, thirty squares of five different colors are assembled. This is a logic puzzle that touches on aspects of mathematics involving both geometry and combinatorics (described below). It is a great exercise in problem solving in which students get to use spatial reasoning to discover and extend geometric and coloring patterns. When complete, students will have created a colorful orb which they can take home or use to decorate the classroom. There is also a nice visual surprise in which students will suddenly see the result in an entirely different manner.

The Paper Square Ball is a wonderful activity for middle and high school students to develop concrete and mental visualization. Furthermore, it works as a team-building project, encouraging collaboration and mathematical communication. With middle school students, we recommend the simpler Paper Triangle Ball beforehand.

This a great example of modular kirigami (assembling cut pieces of paper) and a perfect preparation before more complex paper constructions. In general, explorations with paper can be mathematically rich, inexpensive, and accessible ways for students to start thinking three dimensionally.

Be warned that this activity is trickier than it looks! It will be most successful if the teacher has mastered the steps ahead of time so he or she can demonstrate it with ease. Before attempting this construction, students should have built all five Platonic solids, to be familiar with their symmetries.

This activity provides rich classroom material for teachers following the Common Core Standards for Mathematical Practice. This lesson also provides cross-curricular connections to art, architecture, and higher-level strands of mathematics.

Detailed Instructions

Time Required: 1 Hour

Materials

Card Stock in five colors (one sheet in each of the five colors per pair of students)
Scissors
Template (pdf file)

Notes

Copy the template onto the card stock ahead of time. Only card stock will work. Ordinary copy paper is too thin. Card stock is a heavy weight paper, stiffer than standard paper, but thin enough to snake through the rollers of a copy machine or laser printer. Most copy shops have a selection of colors on hand that they can copy on to for you, or you can buy it by the ream to put in your own copier.
Each Paper Square Ball is made of thirty squares. There are six squares on the template sheet, so five sheets are required to make one orb.
This workshop can optionally be simplified by eliminating the color aspect and printing the template onto one solid color of card stock.
The activity works best when students work in pairs or at most in groups of three. It can be adapted for students to work individually, but would require extra time.
If you have access to a bulk paper cutter (typically at a print shop), it can be used to cut out all the squares at once using this template with registration marks, sent to us by Kathy Lin of the Proof School. This leaves just the diagonal slots to be cut with scissors.

Steps

1. Hand out scissors and the printed card stock sheets. Each group of students receives five sheets---one in each of the five colors.

2. Tell students to cut out the thirty squares and individually cut the four slots in each. Neatness counts! Warn students NOT to stack the sheets or the squares, trying to save time and cut through several sheets at once; that would be too inaccurate.

3. After most groups have cut out at least ten squares, tell students that we will begin exploring the shape now and that they can finish cutting later. Pose the following puzzle, adding these hints gradually, giving students time at each stage:

Find a natural way for three squares to join together. Use three different colors.
Use the slots somehow. Make sure they are slid together all the way.
The result has 3-fold symmetry: A goes to B, B goes to C, C goes to A.
The pieces need to bend slightly when they are being connected, but then are flat after they are joined.
A long slot and a short slot always connect. (Never long to long. Never short to short.)
When done correctly, the result has the appearance of three squares passing through each other.
There will be a small three-color triangle at the center of the joint.

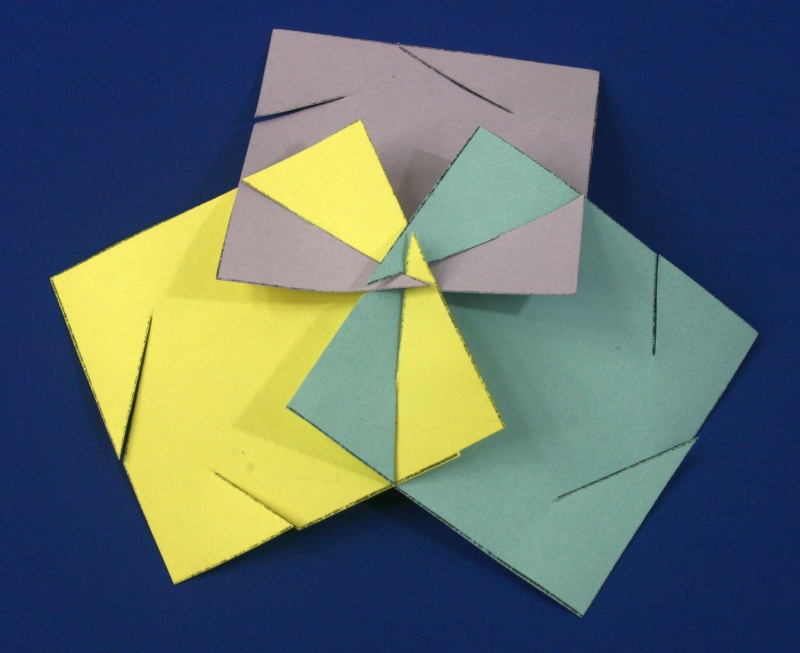
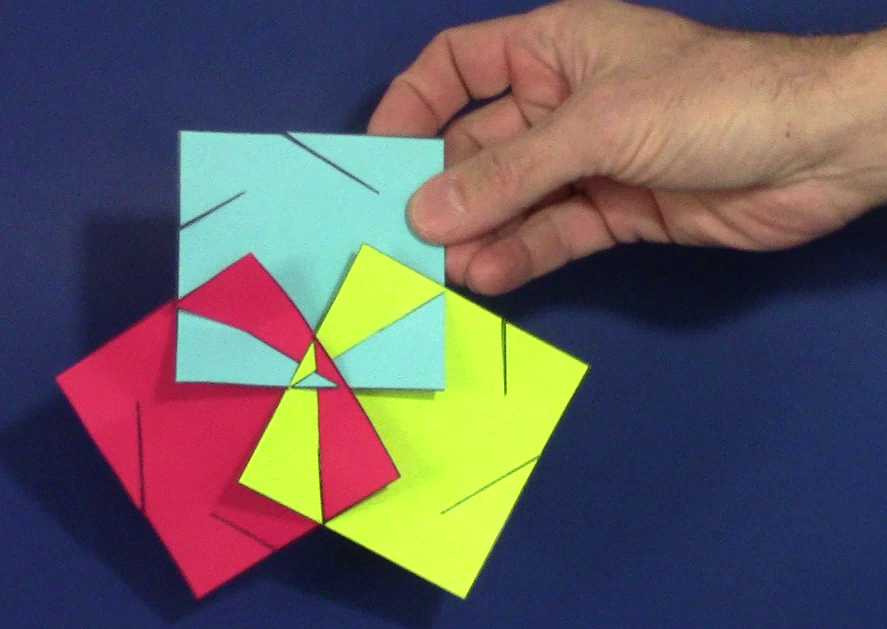
Encourage students to hold up and share their discoveries, giving more hints as needed. Eventually a group will have the correct assembly or you will show them the following 3-square assembly:

4. Hold up a 3-square joint and give the following instructions so every group can replicate it. First observe that the four slots in any square alternate long-short-long-short around the perimeter. The tip of the long slot has a more acute angle than the tip of the short slot. The goal is to bring three of those acute tips together to form a triangle (one from each of the three squares). Join a long slot of one square to a short slot of another square and you will see that two of the acute tips are near each other.

Then with two more connections, you can bring a tip of the third square to the same area. Usually when the third square is connected, the center of the unit has some of the tips bent or hidden underneath other parts. With a small adjustment the three acute tips can be positioned to form a small equilateral triangle in the center of the construction. This small equilateral triangle takes some practice to find, but it is important because it is what locks everything together.

This video may help clarify the process:

Although it takes some time to understand this three-way joint, this is the only type of connection needed anywhere in the Ball. It is easier to do than explain in text. Typically some students discover it then demonstrate it to their peers.

5. Geometrically, the rest of the construction consists of adding one square at a time to the growing structure, making these three-way joints and and being careful to close a 5-way cycle around a pentagonal opening whenever there is an opportunity. The pictures above are a guide for the teacher. In class it is more fun if the students do not have a model to copy. Warn the students not to make 4-sided or 6-sided openings, only 5-sided openings. Warn the students not to try to put together two halves, as the separate color patterns will not be compatible. Instead they need to keep adding one square at a time to one growing structure. Students will discover that some of the edges of the squares form 5-pointed stars (pentagrams) around the pentagonal openings.

6. The color aspect needs to be carefully explained. Before connecting a square, students need to think about what color square can fit there, consistently with the following two rules:

Each square connects to four other squares with its four slots. They must be chosen to be the four other colors of the five. For example, if a square is Red, it might have one neighbor each of Blue, Yellow, Green, and Orange. It should not touch another Red square. It should not touch two Yellow squares.
Each five-sided opening should be surrounded by all five colors.

If students follow these two coloring rules, they will be able to complete the Ball in a consistent way.

7. Walk around the room checking how the constructions progress, helping students and pointing out any problems as early as possible. A common problem is not sliding the slots completely into each other. Be sure always to keep the corners of the squares on the outside of the construction, so the boundary of each square is completely visible. Double check as you go along that every pentagonal opening is surrounded by five different colors and each square joins with four other squares of the four other colors. Keeping the squares flat (planar) and having the corners meet crisply is the key to producing a neat geometric impression.

8. When complete, ask students to examine their Paper Square Ball more closely to see if it reveals any underlying structures they haven't yet discovered. If they need a hint, tell them to look at just any one color. They should see that the six red squares are arranged as an exploded cube. Similarly, the six squares of any other color are arranged as an exploded cube. In fact, the structure can be understood as a compound of five interwoven cubes.

9. You can conclude by showing this video illustrating a family of related paper constructions that might inspire students to explore further.

Conclusions and Extensions

10. Ask students to examine the color pattern on each other's Paper Square Balls to determine whether all the balls in the room are identical. Are there many different color patterns? They should find that half the time they can take any two balls and rotate one so it exactly matches the other in all thirty squares. In fact, there are only two possible legal colorings as explained below.

11. You can ask: How many “three-way corners” are there? (Answer: 20, they correspond to the 20 faces of a regular icosahedron. One way to count them is based on the fact that each of 30 squares touch two three-way corners, and it takes three such contacts to make each, so 30 × 2 / 3 gives 20.) How many 5-sided openings are there? (Answer: 12, corresponding to the 12 faces of a regular dodecahedron, calculated as 30 × 2 / 5.) How many 5-fold rotation axes are there? (Answer: 6. One connects the centers of each pair of opposite 5-fold openings.)

12. You can discuss the combinatorics of the colors meeting at the 3-way joints. Given five colors, ABCDE, how many ways can you choose a subset of three? Answer: ten. ABC, ABD, ABE, ACD, ACE, ADE, BCD, BCE, BDE, CDE. Students can be led to observe that each of those ten combinations happens exactly twice on their Ball. The two positions will be exactly opposite and in reverse order, e.g., if one corner shows ABC in clockwise order, then opposite it will be ABC in counterclockwise order.

12. Similarly, you can discuss the combinatorics of the colors surrounding the pentagonal openings at the high school or higher level. How many different cycles of five colors are possible around a five-sided opening? (Answer: 24, which is 5!/5 because there are 5! permutations of the colors, then “equate” groups of five that are cyclic rotations.) How many different cycles are present in one model? (Answer: 12, one around each of the 12 openings.) So how many differently colored models are in the classroom? (Ans: 2—If the order of initial cycle of five colors is chosen randomly, roughly half the class will have one coloring pattern and half will have the other.) What determines which 12 of the 24 possible cyclic orders are found in the same model? (Answer: The “even” permutations of the five colors are in the same model. The odd permutations appear on the other ball.)

Large-Scale Constructions. After practice with these melon-sized models, the idea can be applied at a larger scale. Large cardboard versions about five feet in diameter can be made from sheets of cardboard.

Related Constructions. For additional similar activities, there are six other slide-togethers in this reference:

G. Hart, "Slide-Together Geometric Paper Constructions," in Adventures on Paper, Math-Art Activities for Experience-Centered Education of Mathematics, Edited by Kristóf Fenyvesi, Ilona Oláhné Téglási and Ibolya Prokajné Szilágyi, Publisher: Eszterházy Károly College, Eger, 2014. (online copy)

The triangles or hexagons are simplest to build. The later ones, with decagons, pentagons, decagrams, and pentagrams are increasingly more difficult. One strategy is to have everyone in a class make a single model and then have different teams each work on a different one of the remaining models. Assign the more difficult ones to the teams which want a greater challenge. Combining the results can make a very attractive display.

References

Charles Butler first described this design to us.

Portions of this material appeared in the 2004 Bridges Conference booklet of Teacher Workshop materials.