Making Math Visible

Six-Pentagons Polylink



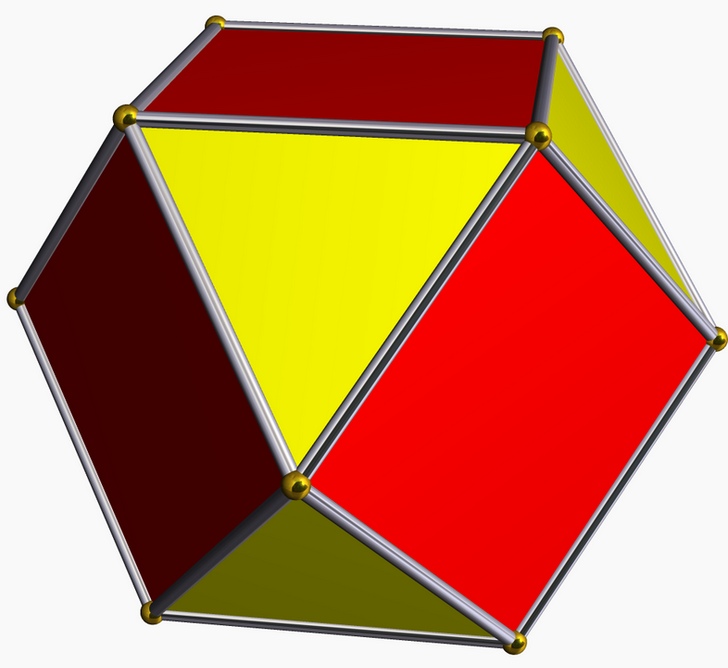
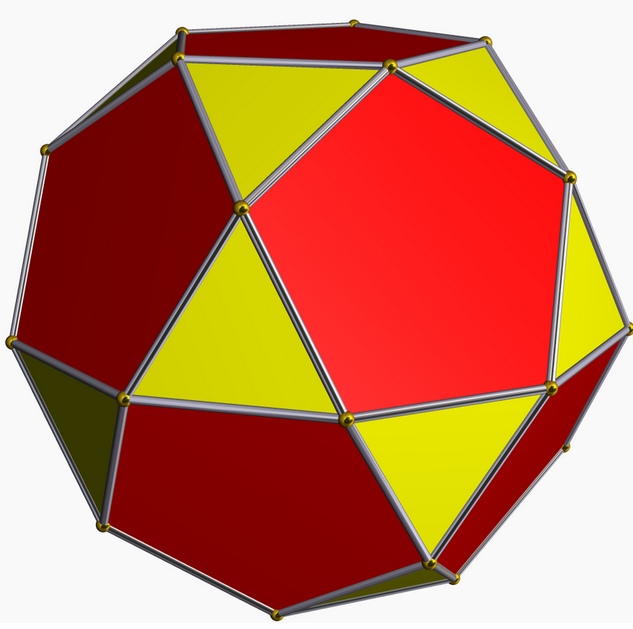
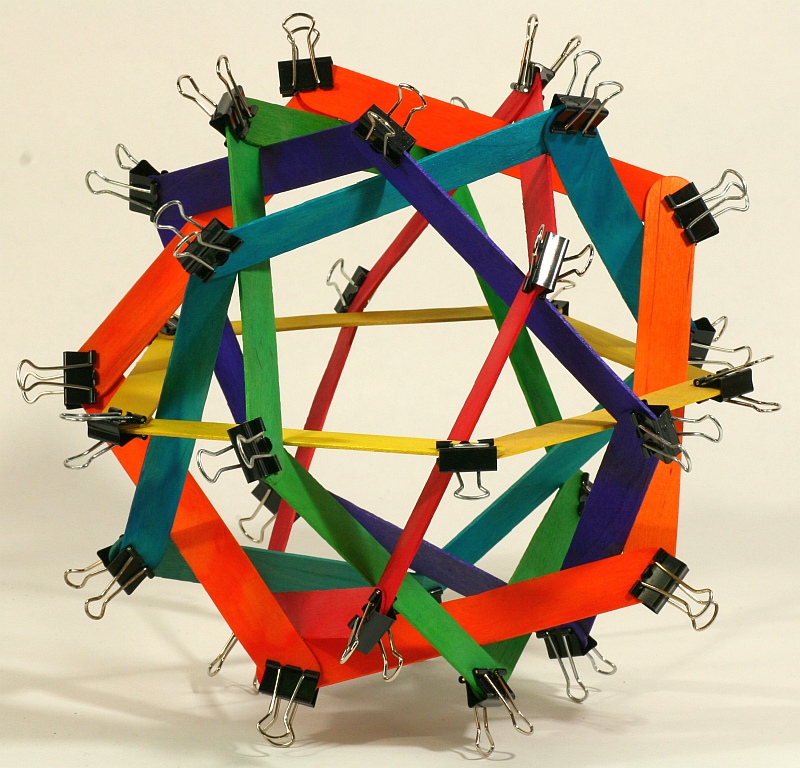
This symmetric arrangement of six hollow pentagons is a harder challenge after the four-triangles polylink and the six-squares polylink, which introduce many prerequisite ideas.  Having 5-way openings, the 108-degree vertex angles of pentagons, and six polygons to interweave, this is a rather challenging puzzle, but the result is a lovely symmetric structure worth knowing.  Again, we present it as a puzzle and don't try to give detailed step-by-step instructions.  We believe that developing the perseverance to find one's own solution is a big part of the value of the activity.

Time Required: 60 Minutes

Materials:
 Notes:

1.  This is the third and most difficult in a series of three polylink workshops. Do the others first as warm-up.
2.  You can use uncolored sticks if necessary, but the result looks much better in six colors.
3.  Working in groups of two is highly recommended.  Students will discover that having four hands is valuable when assembling the parts.
4.  It is helpful to have a model of an icosidodecahedron, e.g., from Zometool,  Polydron, or paper.

Preparation

The 6-inch by 0.7-inch "jumbo craft sticks" we find in the North America are just the right size for this construction, without any need to trim them.  Furthermore, they are available in packs of six colors, exactly what is needed!  If your sticks have a different length-to-width ratio, they may need adjusting analogous to the way the stick ends were trimmed for the triangle or square polylinks.

Part A. Minds-On

  

1. Ask students to recall the discussion of how the four-triangle polylink sits inside a cuboctahedron, shown at left above.  One way we could have derived that polylink is to start with a cuboctahedron, notice it has four equatorial hexagons, and convert each hexagon to a triangle by connecting every other vertex.  You can do this in a consistent manner so each of the original vertices is used in exactly one triangle.  In an analogous way, if you start with an icosidodecahedron, shown at right above, we can derive a new polylink.



2. Show this 1-minute video which illustrates the derivation. Point out that the icosidodecahedron has six equatorial decagons.  (Two cross at each of the 30 vertices.)  We can convert them to six pentagons by taking every other vertex in a consistent manner, with the edges weaving over and under each other.  Analogously to the four triangles, every pair of pentagons is linked and the inner vertices contact the edge midpoints.  The triangular and pentagonal openings of the polylink correspond to the faces of the original icosidodecahedron.  Ask students to try to visualize this and plan how to build it from sticks. 

Part B. Hands-On

1. Divide the class into groups of size two.  Hand out thirty sticks (five in each of six different colors) and thirty clamps to each group. 

 

2. Discuss how to build a regular pentagon.  Somehow a 108 degree angle must be made.  Students can do this accurately enough without a protractor by doing a bit of adjustment and checking.  It is easy to see if a pentagon is regular: Use it as a guide to make a copy of itself (as a second layer on top of it), then rotate the copy a fifth of a revolution.  If the two pentagons always line up, all five angles must be equal.  If not, adjust them a bit and try again.  Use one of these pentagons as a guide for making the remaining pentagon angles.

3. Give students the puzzle of building the six-pentagon polylink they saw in the video, with each pentagon a different color.  This will develop visualization, dexterity,  teamwork, and persistence.
 
4. If requested, play the video again to help students understand the desired structure.


5.  It may take a while for students to find a correct assembly, as shown above.  When a group finishes it, ask them to check: (1) every inner vertex touches some edge and every edge touches some inner vertex.  (2)  The corner overlaps are made in a consistent rotational direction.  (3) Everything is snug with no play between the pentagons.

6. Students who successfully finish their construction can help other groups.

7. The final step will be to glue the joints so the clamps can be removed.  First, ask students to snug up any looseness in their model.  If any movement is possible, a clamp can be removed, the sticks that meet there can be adjusted to be closer together, and the clamp can be replaced.  Do this everywhere until all the joints are snug and there is no relative motion possible between the pentagons.

8. Squirt some wood glue on a scrap of cardboard as a palette.  One at a time, remove a clamp, use a small brush to apply a thin layer of glue in the joint, and replace the clamp making sure the joint is snug.  Tell students not to apply too much; it shouldn't drip out. To keep track of what has and hasn't been glued, it is easiest to do all joints of one color, then all of a second color, etc.

9. Wait 20 minutes or more for the glue to dry before removing the clamps.  While the glue is drying, you can ask the class to ponder whether all the structures in the room must be the same or whether there might be more than one possible solution to the puzzle.


Part C. Conclusion

Ask students to observe the structure and discuss what they discover.  This helps develop a deeper understanding and the ability to visualize it.  Some observations you might elicit are:

1. The six pentagons are concentric.

2. Every pair of pentagons is linked.

3. The five inner vertices of any pentagon are filled with an edge midpoint from the other five pentagons.  The five edge midpoints of any pentagon each sit inside an "elbow" from the other five pentagons.

4. You can stand it up on three vertices in twenty ways, corresponding to the twenty triangles of an icosidodecahedron.

5. You can rest it on five vertices in twelve ways, corresponding to the twelve faces of the icosidodecahedron.  One of the pentagons will be horizontal.

6. It is not the case that if you choose any set of three colors and stand it on three vertices of those colors.  Only some of the triples appear together.

7. The structure can be made in either a left-handed or right-handed manner.  So ignoring color, there are two solutions.

8. For older students interested in combinatorics, one can show there are 12 possible ways the colors could have been arranged, so there are twelve different solutions if you count color for each of the two geometric handednesses.  To see this, note that using six colors, for one cycle of five colors around a 5-way opening, there are 6 ways to omit one color and 24 ways to make a cyclic permutation of the five selected colors.  So there are 144 different 5-way cycles possible.  If a 5-way cycle in one model matches any 5-way cycle in another, then the two models are identical everywhere.  Twelve different 5-way cycles of the 144 appear on each model.  So there are 12 possible models.

9. By relating the shape to a dodecahedron, one can show that no matter which pair of pentagons you choose, the angle between their planes is always the same, roughly 62.5 degrees, which is the angle between the planes of two adjacent faces in a dodecahedron.

 
Possible Extensions

1. Make the mirror-image version to the one you made.

2. Make a giant version from cardboard, thin wood, or other suitable material.



3. Find pictures of the ball used in the game sepak takraw---a traditional sport popular in Asia, something like volleyball in that there are two teams on opposite sides of a net and like soccer in that you don't touch the ball with your hands.  Note how the sepak takraw ball design is isomorphic to the six pentagon polylink.  Weave a ball of this design from the material of your choice.  People have been weaving them for hundreds of years!

4. Many other polylinks are possible.  Watch this video survey and choose some others to build.